— DEV — Strain effects in freestanding nitride nanostructures
Attention
This tutorial is under construction
- Input files:
Strained-QW_AlGaN-GaN_Povolotskyi_PSS_2005_3D_nnp.in
- Scope:
This tutorial aims to simulate strain effects in a lattice mismatched, freestanding heterostructure with wurtzite crystal structure consisting of an AlGaN/GaN quantum well. This tutorial is based on [Povolotskyi2005].
- Output files:
Strain/strain_simulation_2d_slice_middle_along_yz.vtr
Strain/hydrostatic_strain_2d_slice_middle_along_yz.vtr
Strain/strain_simulation_2d_slice_boundary_along_xz.vtr
Strain/elastic_energy_density_2d_slice_middle_along_yz.vtr
Structure
Figure 2.4.146 shows the AlGaN/GaN/AlGaN quantum well structure, which is simulated in this tutorial. A 4 nm wide GaN QW layer is embedded between two
The calculated strain pattern of this AlGaN/GaN structure is found to be highly non-homogeneous. The elastic energy has been minimized using continuum elasticity theory. We assume that the external stress applied to the structure is zero (freestanding structure).
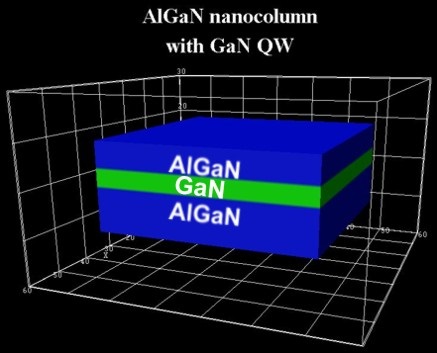
Figure 2.4.146 Simulated heterostructure consisting of a GaN (green) layer sandwiched in between two AlGaN (blue) layers.
Results
Strain tensor components
In this section we show several strain tensor components
Figure 2.4.147 shows the strain tensor component
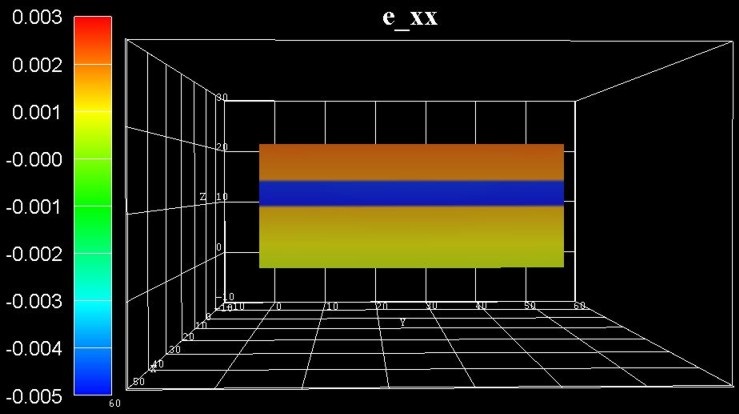
Figure 2.4.147 Calculated strain component
Figure 2.4.148 shows the strain tensor component
In the center (blue region), the GaN takes on the lattice constant of AlGaN (compressive strain).
At the QW boundaries, the GaN takes on the lattice constant of ~GaN (nearly fully relaxed).
Below and above the QW boundaries (red regions), the AlGaN takes on the lattice constant of ~GaN (tensile strain).
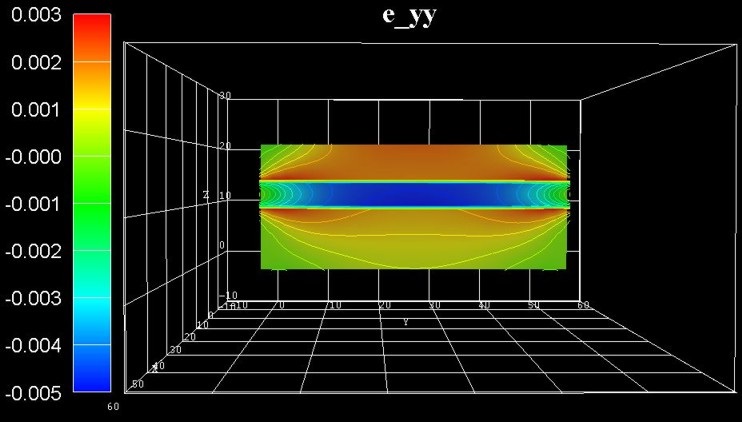
Figure 2.4.148 Calculated strain component
Figure 2.4.149 shows the strain tensor component
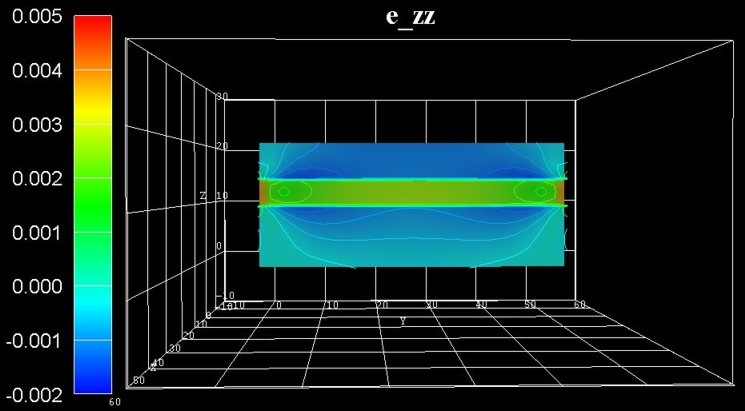
Figure 2.4.149 Calculated strain component
Figure 2.4.150 shows the hydrostatic strain

Figure 2.4.150 Calculated hydrostatic strain component
In contrast to heterostructures, which are infinitely large and homogeneous in the lateral directions (i.e. in the
Figure 2.4.151 shows the off-diagonal strain tensor component
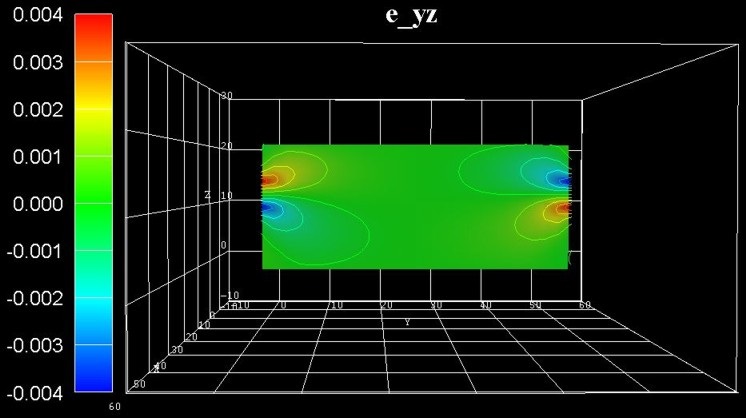
Figure 2.4.151 Calculated strain component
Figure 2.4.152 shows the same off-diagonal strain tensor component
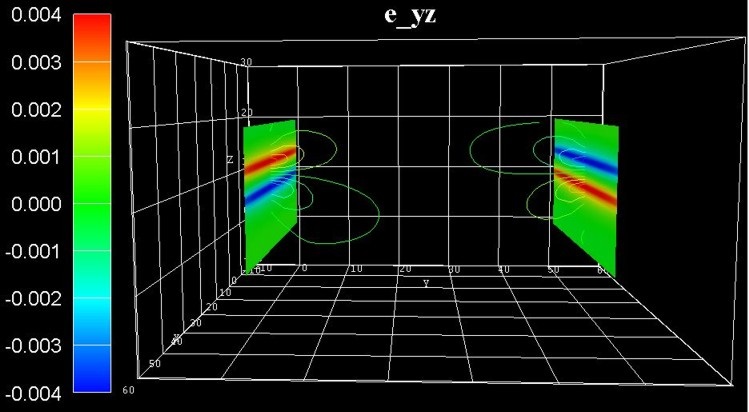
Figure 2.4.152 Calculated strain component
Elastic energy density
Due to a possible usage of such structures as a light emitter, the strain in the GaN layer where charge carriers are confined, is or particular interest, i.e. the influence of stain on the conduction and valence band structure through deformation potentials. Additional, piezoelectric and pyroelectric fields have to be taken into account. The piezoelectric fields depend on the strain distribution in the sample. Thus, both the piezoelectric field and the GaN energy gap will vary along the lateral direction.
Figure 2.4.153 shows the energy density of the elastic deformation in units of
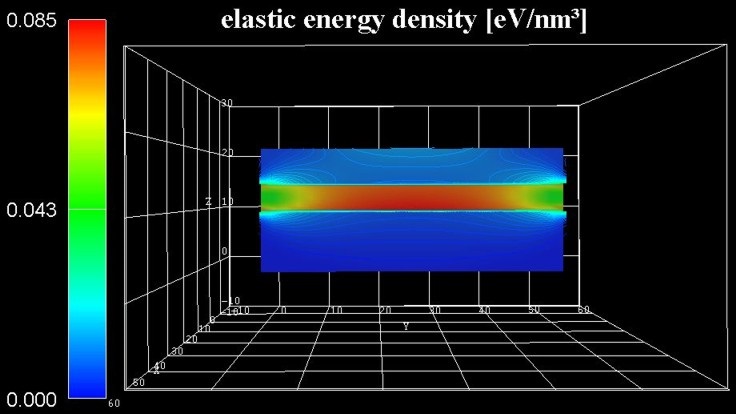
Figure 2.4.153 Elastic energy density in the
Last update: 17/07/2024